Three-Phase Power Calculations

Figure 1: Three-phase power calculations
entail summing of the individual
phases's power calculations

Until now, our discussions of power calculations have encompassed only single-phase systems with one voltage and one current. Now we'll turn to three-phase systems, which can be thought of as a collection of three single-phase systems. In general, three-phase power calculations are a simple summing of the individual phase power calculations and should be balanced across all three phases.

Figure 1 provides a visual representation of how this works. The formulas for real, apparent, and reactive power are:

• Real power (P): P_total = P_A + P_B + P_C
• Apparent power (S): S_total = S_A + S_B + S_C
• Reactive power (Q): Q_total = Q_A + Q_B + Q_C

Figure 2: Line-to-line voltage
measurements as shown above
must be corrected for
magnitude and phase

Simple enough. However, there are some exceptions to the above. For example, if you make line-to-line voltage measurements, the voltages and currents are out of phase and must be corrected for. If your circuit has a Delta winding, you will have a terminal current and not a coil current. Measurements can still be accurate, but you may not have a balance across all three windings. An alternative approach is to use the two-wattmeter method, which will provide the correct total values but only show values for two of the three phases, and those two values will not be balanced.

Three-Phase Line-to-Line Voltage Measurements

Let's look more closely at the line-to-line exception. As mentioned above, in such cases, the voltage and current will be out of phase, so you need to correct for that by doing a magnitude and phase conversion to a line-to-neutral basis. Referring to Figure 2, the mathematical relationships between V_A-B and V_A-N are:

Figure 3: Voltage is often measured
line-to-line but current is measured
line-to-neutral

• Magnitude: V_A-B = V_A-N * √3
• Phase: V_A-B = V_A-N - 30°

These relationships hold true for all three phases in a three-phase system. Perhaps you have a system that has voltages and currents in reference to each other (Figure 3). Voltage is often measured from line to line, but often the neutral point is inaccessible, or you may simply prefer line-to-line probing. Meanwhile, current is measured on a line-to-neutral basis. So line-to-line voltages must again be transformed to a line-to-neutral basis. We make the calculations as shown above and sum the phases to find totals in each case.

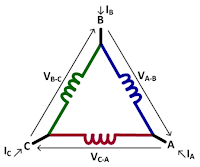Figure 4: With Delta windings,
power balance across all three phases
may be elusive in calculations

The Delta-Winding Exception

In situations involving a Delta winding, voltage is still measured line-to-line: It has to be as the neutral point is inaccessible. The current to be measured is the terminal current, which is not the same thing as the "coil" currents. The calculations as shown above will still be correct, but you may not have power balance across all three phases because you're not really measuring the "coil" current. Still, total three-phase power is still calculated as described above by summing the phases.

Two-Wattmeter Three-Phase Power Measurements

A two-wattmeter power measurement can be performed on a Delta or Wye winding (Figure 5). In either case, voltage is measured line-to-line on two phases, with both referenced to a common point. In the examples shown in Figure 5, the measurements are VA-C and VB-C, with C being the common reference point. Current is also measured on these two phases, so we have the A current and B current both flowing into the neutral point C.

Figure 5: The two-wattmeter method of making three-phase
power measurements works on either Delta or Wye
windings as shown

This method works because we can make mathematical assumptions. If we know two of the three voltages and currents, we can solve for the third. According to Thevenin's Theorem, we can take a line-to-line voltage and a line current, and a line current and a line-to-line voltage, and calculate the three-phase power directly from that information. The mathematical assumptions are as follows:

• Σ(I_A + I_B + I_C) = 0
• Σ(V_A-B + V_B-C + V_C-A) = 0

Our final post in this series will tie everything together by reviewing an example of a three-phase power measurement from a 480-V motor drive.

Previous posts in this series:

Back to Basics: The Fundamentals of Power
Back to Basics: Fundamentals of AC Line Power (Part II)
Back to Basics: Three-Phase Sinusoidal Voltages
More Basics of Three-Phase Sinusoidal Voltages
Back to Basics: AC Sinusoidal Line Current
Power Calculations for Pure Sine Waves
Power Calculations for Distorted Sine Waves